Figuring and Testing The
Mirror
Making a 4.25 Inch Dobsonian
Reflector Telescope
Testing
Once your mirror is polished, you need
a way to test it in order to adjust or figure the mirror. Figuring is done by polishing the mirror in
a way that guides it toward an optically perfect parabaloid. At this stage, the size of the mirror and
the F number will become important. If
you have a small mirror and/or a large F number, your job may already be
done. There are a few different ways
to test your mirror.
The
Star Test
To do a star test, you do not actually
need a star, however you will need to complete the assembly of your
telescope. This is because you will
need to point it at something in order to evaluate the image you are
seeing. Instead of a star, you can
point your scope at a telephone pole insulator that is positioned to reflect
the sun. You may also use a bright
star. The technique is to defocus the
telescope and look at the fuzzy disk.
If the disk is uniform, then you have a fairly well figured mirror. If it is uneven, the location of the dark
and light rings tell you what you need to do to correct it. I cannot tell the details. I think this is a reasonable method for a
small mirror or one with a large F number.
The
Ronchi Tester
A Ronchi tester is very similar to the
Foucault tester that I will describe below.
The difference is that instead of a knife edge, the Ronchi tester uses a
grating. The image you get consists of
curved dark and light lines on the surface of the mirror. The catch is that you need to know what
those lines should look like for your specific mirror. I'm told that there is free software on the
web to answer this question.
A
Ronchi Eyepiece
Yes, you can buy an eyepiece with a
grating. While probably not as
sensitive as a Ronchi tester, there is one advantage to using an eyepiece. You are looking at a star at infinity so
the pattern you are looking for is always the same, straight parallel
lines. I think this method is
probably as good as the star test, at the disadvantage of needing a special
piece of equipment.
The
Foucault or Knife Edge Tester
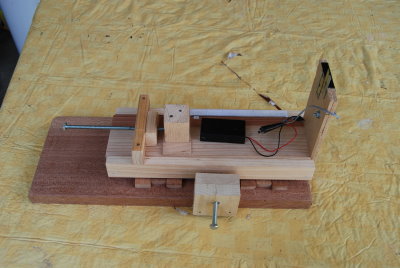
Building your own Foucault tester is
not all that difficult. There are many
designs you can find on the web. The
basic tester needs two axes to move a small table. The forward/backward movement is crucial. Usually a long bolt with threads of known
size is used. By turning the bold, you
can move the table a very precise known distance. The other axis is used to move back and forth to find a null
point. Other components you need are
an LED for illumination and a straight edge razor for the knife edge.
The
Theory
I'm only going to give a brief
overview here. I recommend you check
out a good resource on this subject.
One I found useful was "Understanding
Foucault" by David A HarBour.

Consider
what would happen if you had a pin point light source at the focal point of
your spherical mirror. The light from
the pinpoint would bounce back from the mirror and cross at the original
source. Now if you were to move this
pin point slightly off center, the light would again converge at a point, but
one that was accessible. Now imagine
you are trying to find this point by standing behind it, and placing a knife
across the light path. If the knife
edge is between you and focal point, you will see a dark shadow cross in the
same direction as the knife edge is moving.
If the knife edge is behind the focal point, you will again see a
shadow, but it will move in the opposite direction. So by moving the knife edge in and out, and then running it
across your sight line, you can find the focal point. The closer you are to this point, the faster the shadow
moves. When you are very very close to
the point, instead of seeing a shadow, the image of the mirror surface will go
dark all of a sudden.
That's
the basic idea of finding the null point of a spherical mirror. The catch is, you don't want a spherical
mirror, you want one in the shape of a parabaloid. Remember that the sphere and the parabola are already very
close to being one and the same. You
can model a corrected parabaloid by thinking of the mirror as divided into a
number of rings called zones, which each is a sphere, but at a slightly
different focal length. You put a mask
over the mirror called a Couder mask, seen in the photograph above, and then
find the null point for each zone.
There is free software available on the web to create Couder masks.
Having
taking careful measurements, you now can compare them to a set of calculated
measurements. The difference tells you
which rings of the mirror need to be worn down more by polishing.